A function f is said to be discontinuous at a
point a of its domain D if it is not continuous threat. The point a is then
called a point of discontinuity of the function. The discontinuity may arise
due to any of the following situations:
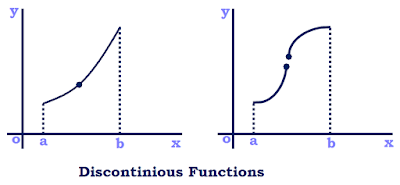 a)
a)  or
or  of both may not exist.
of both may not exist.

 or
or  of both may not exist.
of both may not exist.
b)  as well as
as well as  may exist, but are unequal.
may exist, but are unequal.
 as well as
as well as  may exist, but are unequal.
may exist, but are unequal.
c)  as well as
as well as  both exist, but either of the two or both may
not be equal to f(a).
both exist, but either of the two or both may
not be equal to f(a).
 as well as
as well as  both exist, but either of the two or both may
not be equal to f(a).
both exist, but either of the two or both may
not be equal to f(a).
We classify the points of
discontinuity according to various situations discussed above.
1. Removable discontinuity: A function f is said to have removable
discontinuity at x = a if  but their common value is not equal to f(a). Such
a discontinuity can be removed by assigning a suitable value to the function f
at x = a.
but their common value is not equal to f(a). Such
a discontinuity can be removed by assigning a suitable value to the function f
at x = a.
 but their common value is not equal to f(a). Such
a discontinuity can be removed by assigning a suitable value to the function f
at x = a.
but their common value is not equal to f(a). Such
a discontinuity can be removed by assigning a suitable value to the function f
at x = a.
2. Discontinuity of the first kind: A function f is said to have a discontinuity
of the first kind at x = a if
 and
and  both exist but are not equal. f is said to
have a discontinuity of the first kind from the left at x = a if
both exist but are not equal. f is said to
have a discontinuity of the first kind from the left at x = a if  exists but not equal to f(a). Discontinuity of
the first kind from the right is similarly defined.
exists but not equal to f(a). Discontinuity of
the first kind from the right is similarly defined.
 and
and  both exist but are not equal. f is said to
have a discontinuity of the first kind from the left at x = a if
both exist but are not equal. f is said to
have a discontinuity of the first kind from the left at x = a if  exists but not equal to f(a). Discontinuity of
the first kind from the right is similarly defined.
exists but not equal to f(a). Discontinuity of
the first kind from the right is similarly defined.
3. Discontinuity of second kind: A function f is said to have a discontinuity
of the second kind at x = a if neither  nor
nor  exists.
exists.
 nor
nor  exists.
exists.
A
function f is said to have discontinuity of the second kind from the left at x
= a if  does not
exist. Similarly, if
does not
exist. Similarly, if  does not exist, then f
is said to have discontinuity of the second kind from the right at x = a.
does not exist, then f
is said to have discontinuity of the second kind from the right at x = a.
 does not
exist. Similarly, if
does not
exist. Similarly, if  does not exist, then f
is said to have discontinuity of the second kind from the right at x = a.
does not exist, then f
is said to have discontinuity of the second kind from the right at x = a.
No comments:
Post a Comment